"Посмотрите  что происходит с центропланом после отрыва кокпита, центроплан уходит в крутое пикирование." - это же Ваши слова?
А вот с mh17 вышло по-другому, он еще значительное расстояние пролетел на высоте около 10000. И только потом стал плавно уходить в крутое пикирование.
У Бутблека уходит в пикирование стразу, но потом выполаживается. За что ему попало от Альберта Валиева.

Отредактировано uschen (2021-10-28 22:04:45)

Понятия не имею. Но я придерживаюсь точки зрения, что если неуправляемый летящий объект начал заваливаться вбок и пикировать, он уже не остановится до самой земли. Бумажного самолетика, который снижается волнами, не получится.
А Боинг улетел довольно далеко. Значит он не начал сразу пикировать.
Валиева можно почитать у Бутблека, он с авиасимуляторами связан.
Я не то, чтобы моделирую самолёт. Скорее конкретизирую - если он летел таким вот образом, то это должно было проходить вот именно так.
Ну а дальше можно проверять. Например, есть предельная приборная скорость - посмотреть, стабилизатор разломало, когда она была достигнута?
Если нет - надо править. Если да - косвенное подтверждение, что да, так могло быть.

Отредактировано uschen (2021-10-28 22:33:03)

Учитывая, что шел по дуге - далеко.
Что значит - стал пикировать? - отсутствие подъемной силы, по крайней мере вертикальной составляющей.
Ну так попробуйте камень зашвырнуть в Грабово, вместо подъемной силы в горизонтальном направлении сделайте соответствующий ветер. Посмотрим, как будет выглядеть его путь. По идее - сначала вперёд, а потом вниз и вбок.

oper. а ты не задумывался о том что твой способ определения БК фрагмента по координатам его падения, настолько же... своеобразен как и способ Risto определения БК по уровню перегрузки ELT ?

Он определяет БК по перегрузкам.
Ты определяешь БК по координатам.

Чем вы отличаетесь друг от друга по альтернативности определения Баллистических Коэффициентов объектов ? 

А где я могу взять эти данные ? 
Стартовые условия для фрагментов из "задачи Акулича"  где они в принципе могут находиться ? 

Ну а ты ввёл данные DSB, получил результат ?

Стартовые условия по данным DSB соответствуют действительности ?

Ты можешь при помощи своей программы определить стартовые условия для упавших фрагментов ?

Откуда "упал рубль" можешь определить ?

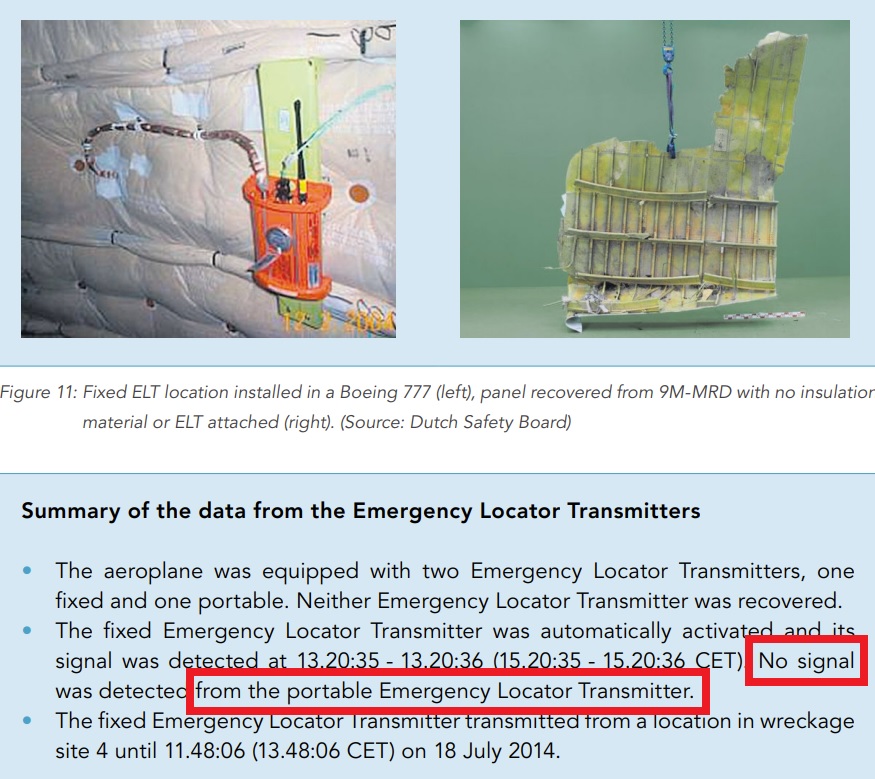
OK, the signal can be from fuselag ELT. But it is no better for Bellingcat / DSB / JIT false interpretations.

If the signal is from fuselag ELT, it does not give the strike moment of the missile, but IT GIVES THE MOMENT WHEN THE LAST MOTOR (on the right side) STOPPED WORKING.

The broken cockpit can have been still connected to the fuselage pressed by the drag force 2.3x10m/s²x10000kg = 230kN = 23Mp = 23 "ton".
The cockpit falls without gliding, but the fuselage has gliding flight in addition to free fall under drag. An undamaged Boeing 787 under guinance can fly in glide flight ten times longer without motors from that height and speed.

This is as valid as before.

The accelerations during explosion are not here useful. Explosion as such did not wake up ELT of longitudinal deceleration (acceleration), it was activated from drag deleleration when when cockpit was disconnected from fuselage. Despite deceleration 2 ... 2.6 g, speeds where measured. We can compare them with theoretical functions:

Time, s    Measured  v, m/s  v´(0) = 2.0 g  v(t) = 3125 m/(t + 12.5s)  v´(0) = 2.6 g  v(t) =  v(t) = 2403m /(t + 9.6s)   v´(0) = 2.3g v(t) = 2717,4m/(t + 10.87s)

   0                 250                                    250                                                           250                                                                                          250,0

   1                 230                                    231                                                           226                                                                                          229,8

   2                 211                                    215                                                           207                                                                                           211,1

According to Newton´s (first and) second law and his drag law, for a body moving in a dragging fluid

v´(t)/v²(t) = constant = v´(0)/v²(0) = 2.3g/vo² = c/m  = 23m⁻¹/250² = (2717,4m)⁻¹, when no extra forces influence.  c =ρAC/2.

mdv/dt = mv´(t) = - ρACv²(t)/2 = - cv²(t) = the drag force

v´(t)/v²(t) = - c/m = constant = -2.3g/vo² = -23ms⁻²/(250ms⁻¹)² = - (2717m)⁻¹ = -k

Integrating implicitely:

-v⁻¹(t) = -k(t - to), where to is integation constant, which gives

v(t) = k⁻¹(t - to)⁻¹

v(0) = 250ms⁻¹ = vo = -k⁻¹to⁻¹, which gives

to = -k⁻¹vo⁻¹ = - vo/(2.3g) = - 250ms⁻¹(23ms⁻²)⁻¹ = - 10.87s.

The distance d(t) at time t:

d(t) = int v(t)dt = k⁻¹[ln (t - to) - ln (0 - to)] = k⁻¹ ln (1 - t/to) = 2717,4m ln(1 + t/11.87s)

(The density of air ρ depends on height: 1n 10000m it is about 0.5kg/m³, on sea level it is about 1.0kg/m³. We use now the former. If we use the latter for the horizontal fall, it means longer fall time T and longer fall flight D.)

When we know a (trajectory) constant in one point of a trajectory, we know it in every point.

In this case we know at the disconnection point t = 0 both the speed v(0) = 250 m/s and the acceleration v´(0) = -2.3g = -23m/s²

In fall falling distance w(t)

m dw(t)dt = mw´(t) = mg - cw²(t),

w´(t)/(w²(t) - mg/c) = c/m = k, which is taken from the former task using lower air ρ = 1.0.

Let´s define still one coefficient:

k/g = p² = c/(mg) = a²/vo², p = a/vo = 116.6⁻¹m⁻¹s

(m in two meanings: the mass and meter...)

w´(t)/(w²(t) - p⁻²) = gp²:

- p artanh (p w(t)) = (t - t1) gp²,

where t1 = 0 from v(0) = 0.

w(t) = p⁻¹ tanh(gpt)

and falling "height" (from the top):

h(t) = k⁻¹ln cosh(gpt)

Отредактировано Risto_Koivula,_Tampere (2021-10-29 22:39:18)